After the second world war the world was left in a precarious state. Two superpowers were jockeying for position, and the stakes were nothing less than world-wide nuclear destruction. A wrong move was not an option, and a branch of mathematics called game theory was developed to study how individuals could best choose their actions. Whether it helped the Cold War end peacefully is debatable, but game theory is still useful in many different fields today. Studying motives and payoffs applies not just to traditional games, but also strategic decisions in economics and business.
Game On
What are the rules, and what’s the best strategy to win? Does it matter what other people do? What are they going to do? Can everyone be a winner, or is any gain going to be at the expense of others? These are the sort of questions which game theorists consider.
In an economic context, the agents making the choices will be companies or even whole countries. Companies are in direct competition and, while they know what their competitors might do there’s no way of knowing what they will do – just the situation game theory was designed for.
Example
Here’s a simple example of a set-up addressed by game theory. There are two rival companies, and one of them is considering launching a new product with a splashy ad campaign which will cost £10 million. But if they do this, they run the risk that the other company might respond with a campaign of their own. With two big ads running at the same time, the campaign will have less influence on consumers.
The options for both companies can be shown in a table called a ‘decision matrix’. This shows the results of choices by either company and how they are affected by what the other one does. Profits (in millions of pounds) for Company 1 are shown in red and Company 2 shown in blue.
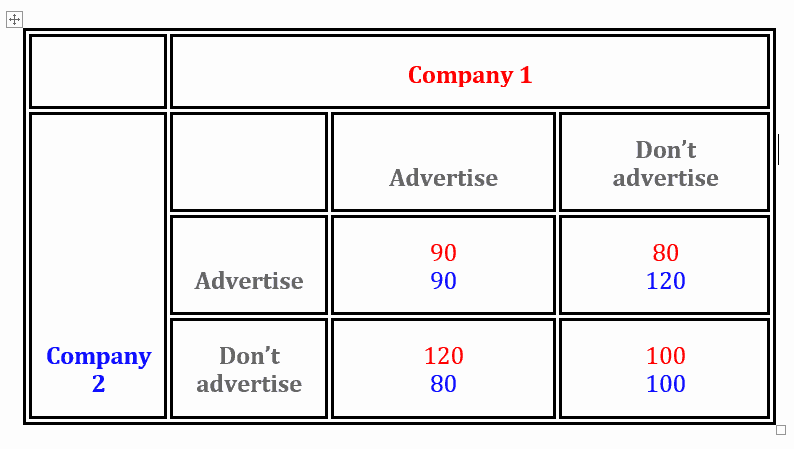
The numbers give the profits that Company 1 and Company 2 respectively would make if they picked that column or row. You can see that a company will do best if their rival doesn’t run a campaign. If neither company campaigns – then profits are split equally between the two. If they both advertise, they make the same money, but they also have to pay for the ad campaign. Which option should they pick?
Decisions Decisions

When Company 1 is trying to choose whether or not to advertise, they look at the options and see that if they advertise, the least they’ll make is £90 million. However, if they don’t advertise, profits could drop to £80 million. Since they don’t know what Company 2 will do, they’re best off choosing the option which is safest – advertising.
Company 2 can also see that they are safest to advertise – they avoid the loss of being the only one without an ad campaign. So independently, both companies will end up with competing ad campaigns and £10 million less than if they had both agreed not to advertise.
This kind of analysis is a branch of decision maths. It’s used by strategists for product pricing, auctions and even negotiating jail terms.
More general information can be found at: gametheory.net






